Question answered
On 10th June, a question was posed:
First, we will plot the graph of v-t:
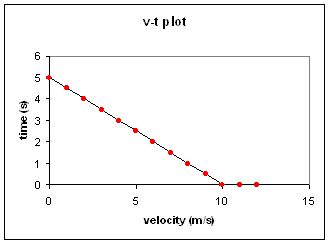
Notice that the ball stops moving completely once it comes to a halt. The v(t) function is piecewise continuous- there is constant deceleration in the first 10 seconds, and everything after that is v=0.
Expressed as an equation:
If v(t) did not hold still at 0 after t=10, it would be a rather disturbing phenomena- a ball rolled along a horizontal floor surface would slow down to a halt, and then speed up in the opposite direction indefinitely.
Thus, after the ball has come to a halt, there will be no further displacement. The total displacement in the first 12 seconds is the same as the displacement in the first 10 seconds.
If we insist on doing the integral:
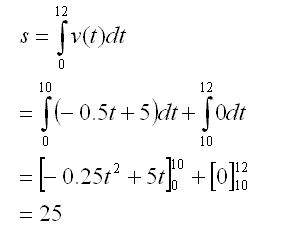
A ball is rolled over a horizontal, rough surface with an initial velocity of 5 m/s. Friction will cause the ball to decelerate at a constant rate of 0.5 m/s2. Calculate the distance travelled by the ball in the first 12 seconds.
Hint: substituting t=12 into s = 0.5at^2 + ut does not work. v(t) is a piecewise continuous function.
First, we will plot the graph of v-t:
Notice that the ball stops moving completely once it comes to a halt. The v(t) function is piecewise continuous- there is constant deceleration in the first 10 seconds, and everything after that is v=0.
Expressed as an equation:
If v(t) did not hold still at 0 after t=10, it would be a rather disturbing phenomena- a ball rolled along a horizontal floor surface would slow down to a halt, and then speed up in the opposite direction indefinitely.
Thus, after the ball has come to a halt, there will be no further displacement. The total displacement in the first 12 seconds is the same as the displacement in the first 10 seconds.
If we insist on doing the integral:
posted by Tan Yee Wei at 6/12/2005 01:41:00 am
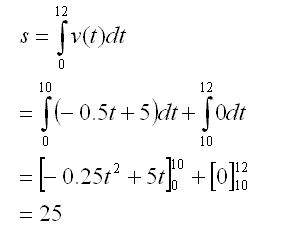
<< Home