e - unifying two common definitions of e
Note: The concept of infinity would be used liberally here. For a brief introduction to the origins of the infinities found in today's context, read this post.
Perhaps the most common expression that gives the value of e would be the sum of the reciprocal of factorials, from zero to infinity.

Equation (1)
Another well known expression is:
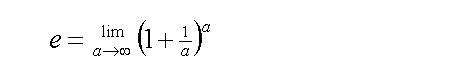
Equation (2)
I will attempt to show that these two expressions are identical. Firstly, I will let a be a finite number before pushing it towards the great infinity.
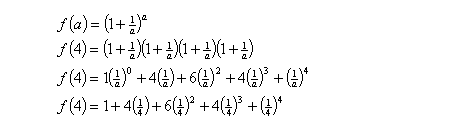
Equations (3)
Here, a takes the value of 4. Expanding the brackets, we see that the terms are results of multiplication between 1 and ¼. In the last line above, the coefficients 1, 4, 6, 4, 1 are actually binomial coefficients resulting from 4C0, 4C1, 4C2… 4C4.
For example, 4C2=6 arises because (¼)^2 appears 6 times as a result of the 6 different ways the terms can combine to give (¼)^2. The combinations are:
If you have no idea what I am talking about, try expanding f(6) into individual terms. It should become clear after that.
f(a) can then be rewritten as
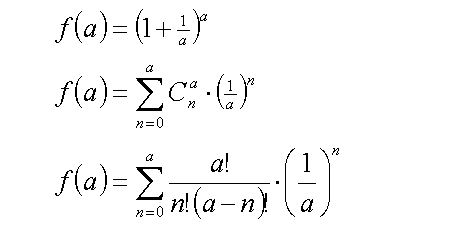
Equations (4)
Expressing the binomial number as a ratio of factorials gives the final line in Equations (4).
Substituting f(a) into the definition of e (equation 2):
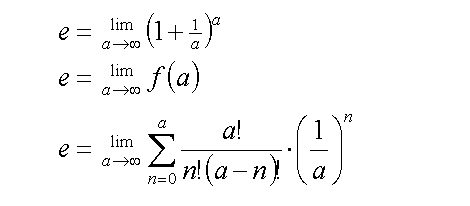
Equations (5)
The equation is beginning to resemble the infinite sum of inverse factorials:
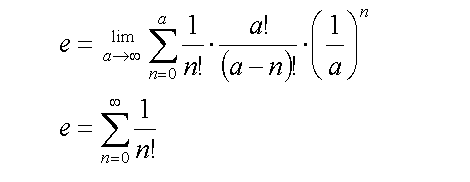
Equations (6)
To show that these equations are equivalent, the following expression needs to be true:
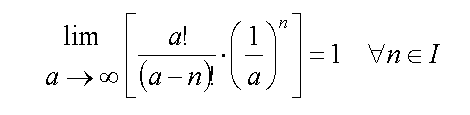
Equations (7)
When a approaches infinity, the terms in the brackets should converge to unity, and the expressions in equations (6) would be identical.
Expanding the terms in the bracket:
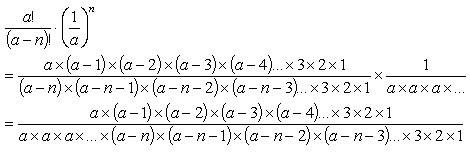
Equations (8)
Cancelling terms that appear in the numerator and denominator:
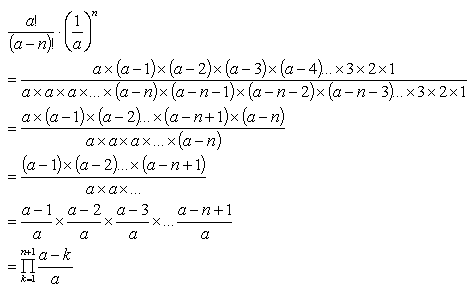
Equations (9)
Note that there are an equal number of terms in the numerator and denominator. For (a-n)!, the missing terms are replaced with the a^n.
Replacing this result into the previous expression:
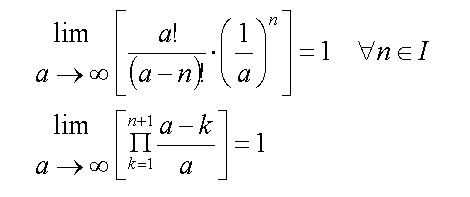
Equations (10)
When taking the limit of a approaching infinity, (a-k)/a is unity regardless of the value of k. After all, infinity minus 5 is still infinity.
Thus, it summary
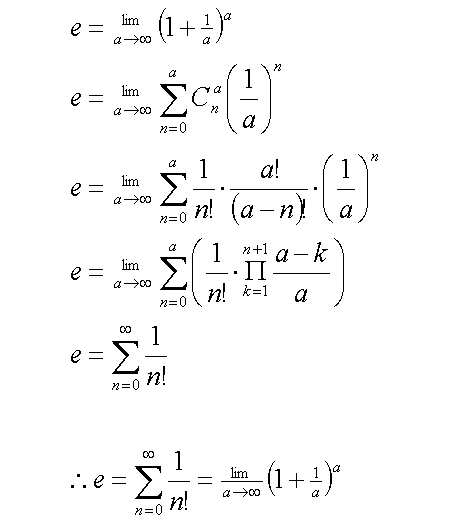
Equations (11)
One very valid concern is, “what happens when k ≈ a? When k ≈ a, (a-k)/a is no longer unity and equation (6) might not match term-for-term.”
Fortunately, this never happens. Observe how the equation is structured: first, let a approach infinity. Then, incrementally increase n and k.
n and k can be as humongous as one wishes to make it, but they would always be dominated by a. You can even make n and k as large as the second Skewe’s number, and they would still not make a dent in a. The second Skewe’s number is finite; a is not.
Perhaps the most common expression that gives the value of e would be the sum of the reciprocal of factorials, from zero to infinity.

Equation (1)
Another well known expression is:
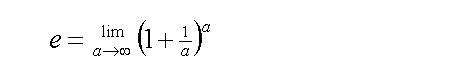
Equation (2)
I will attempt to show that these two expressions are identical. Firstly, I will let a be a finite number before pushing it towards the great infinity.
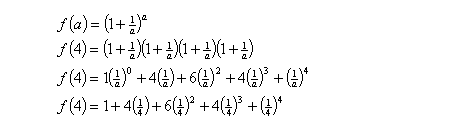
Equations (3)
Here, a takes the value of 4. Expanding the brackets, we see that the terms are results of multiplication between 1 and ¼. In the last line above, the coefficients 1, 4, 6, 4, 1 are actually binomial coefficients resulting from 4C0, 4C1, 4C2… 4C4.
For example, 4C2=6 arises because (¼)^2 appears 6 times as a result of the 6 different ways the terms can combine to give (¼)^2. The combinations are:
Multiply ¼ from the 1st and 2nd brackets, and multiply with 1 from the 3rd and 4th brackets.
Multiply ¼ from the 1st and 3rd brackets, and multiply with 1 from the 2nd and 4th brackets.
Multiply ¼ from the 1st and 4th brackets, and multiply with 1 from the 2nd and 3rd brackets.
Multiply ¼ from the 2nd and 3rd brackets, and multiply with 1 from the 1st and 4th brackets.
Multiply ¼ from the 2nd and 4th brackets, and multiply with 1 from the 1st and 3rd brackets.
Multiply ¼ from the 3rd and 4th brackets, and multiply with 1 from the 1st and 2nd brackets.
If you have no idea what I am talking about, try expanding f(6) into individual terms. It should become clear after that.
f(a) can then be rewritten as
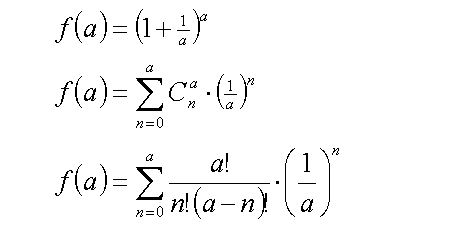
Equations (4)
Expressing the binomial number as a ratio of factorials gives the final line in Equations (4).
Substituting f(a) into the definition of e (equation 2):
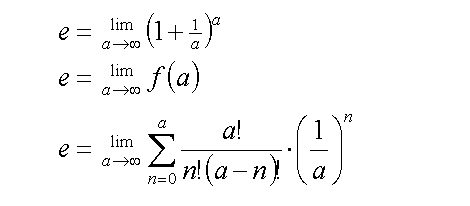
Equations (5)
The equation is beginning to resemble the infinite sum of inverse factorials:
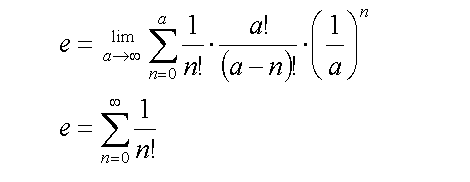
Equations (6)
To show that these equations are equivalent, the following expression needs to be true:
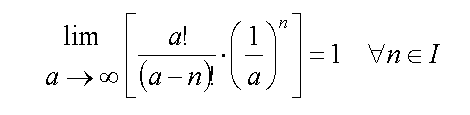
Equations (7)
When a approaches infinity, the terms in the brackets should converge to unity, and the expressions in equations (6) would be identical.
Expanding the terms in the bracket:
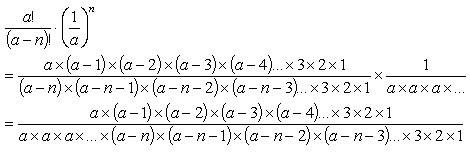
Equations (8)
Cancelling terms that appear in the numerator and denominator:
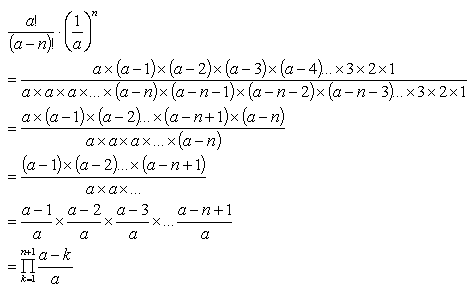
Equations (9)
Note that there are an equal number of terms in the numerator and denominator. For (a-n)!, the missing terms are replaced with the a^n.
Replacing this result into the previous expression:
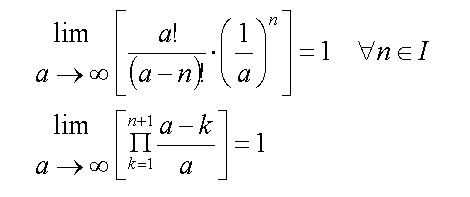
Equations (10)
When taking the limit of a approaching infinity, (a-k)/a is unity regardless of the value of k. After all, infinity minus 5 is still infinity.
Thus, it summary
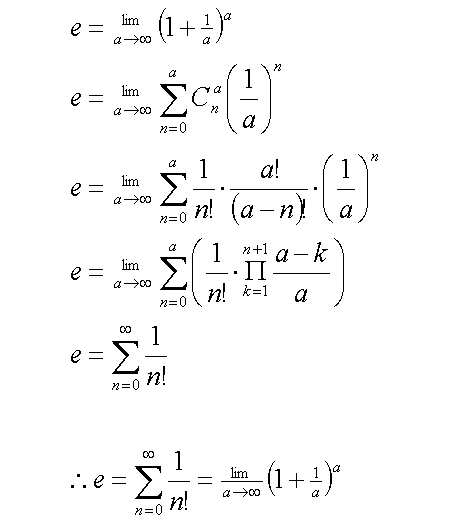
Equations (11)
One very valid concern is, “what happens when k ≈ a? When k ≈ a, (a-k)/a is no longer unity and equation (6) might not match term-for-term.”
Fortunately, this never happens. Observe how the equation is structured: first, let a approach infinity. Then, incrementally increase n and k.
n and k can be as humongous as one wishes to make it, but they would always be dominated by a. You can even make n and k as large as the second Skewe’s number, and they would still not make a dent in a. The second Skewe’s number is finite; a is not.
Labels: mathematics, real numbers

<< Home